RESIDUE THEORY
1.1
Cauchyís Residue Theorem
We now state a theorem of practical importance called the
Cauchy Residue Theorem which allows us to compute
easily many integrals some of which can only be evaluated
using this theorem.
Theorem 1.
(Cauchy Residue Theorem). Suppose that
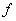 is an analytic function on a simply-connected domain
is an analytic function on a simply-connected domain
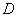 except for a finite number of poles
except for a finite number of poles
 Suppose that
Suppose that
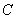 is a piecewise smooth positively-oriented simple (does not
intersect itself) closed curve not passing through
is a piecewise smooth positively-oriented simple (does not
intersect itself) closed curve not passing through
 Then
Then
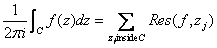 , ,
where the sum is
over all the poles
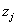 of
of
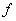 that are inside
that are inside
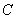
Proof.
By a previous theorem,
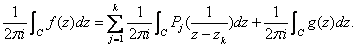
By Cauchyís
Theorem,
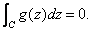 Moreover,
since Moreover,
since
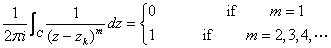
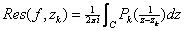
1.2
Jordanís Lemma
In some cases we use the following:
Lemma 1.
(Jordanís lemma). If
 represents the curve
represents the curve
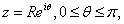
then
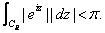
Proof.
On
 , ,
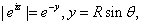 and
and
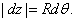 Thus
Thus
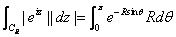

In the last
integral let
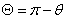 ,
then using ,
then using
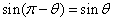 we get,
we get,
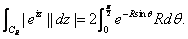 But But
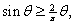 for
for
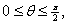 from which the result follows.
from which the result follows.
As an application of Jordanís lemma we consider the
following
Example. Show that
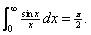
Method :
Since :
Since
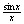 is an even function, it suffices to show that
is an even function, it suffices to show that
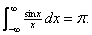 Let
Let
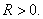 Consider the integral
Consider the integral
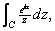 where
where
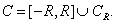 However, since the singularity
However, since the singularity
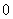 is on the contour of integration, we consider instead the
integral
is on the contour of integration, we consider instead the
integral
 In this case,
In this case,
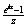 has
a removable singularity has
a removable singularity
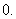 Thus the function
Thus the function
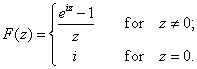
is entire and
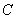 is a closed contour in
is a closed contour in
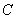 .
Then by Cauchyís Theorem we have .
Then by Cauchyís Theorem we have

Then
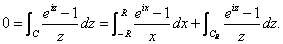
So
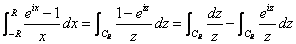
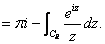
Equating
imaginary parts we get
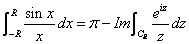
So
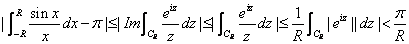
by using
Jordanís lemma.
Finally, letting
 establishes the result.
establishes the result.
Method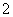 :
Let :
Let
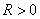 be large and
be large and
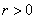 be small. Now let
be small. Now let
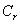 represent the curve
represent the curve
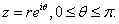
Consider the
integral
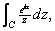 where
where
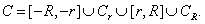 Note that the function
Note that the function
 has no singularities inside
has no singularities inside
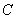 .
Thus .
Thus
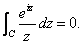
So
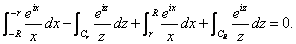
Letting
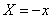 in the first integral we get
in the first integral we get
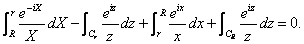
Combining we get
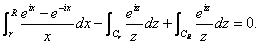
or
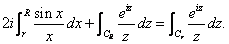
Moreover, near
 we have the expansion
we have the expansion
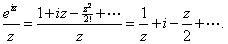
Hence
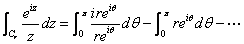
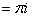 +terms
that tend to +terms
that tend to
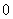 as
as
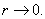
Furthermore, using Jordanís lemma, we get

Finally, letting
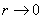 and
and
 establishes the result.
establishes the result. |

